BA PART I : PAPER 3.13

MATHEMATICAL ASTROLOGY

Definitions

Plane:

When a plane surface is extended, it is called a plane. It has got
only length and breadth and not the width. It is a two dimensional
figure. Three points which are not in a straight line, determine a plane.
Its examples are the upper part of the floor, top of the table etc.

Curved surface:
All the points of a curved surface do not lie in a plane for example
surface of a cone, surface of a ball etc.

Circle:
All the points of a circle are equidistant from one point. That
point is the centre of the circle. The distance of centre with any point on
the circle is called radius. A straight line passing through the centre and
meeting the circle at two points opposite to each other is called diametet

Sphere:
If a semi-circle is rotated around its diameter, the three dimensional
figure formed is called a sphere. All the points on the surface of a
sphere are equidistant from the centre and this distance is known as
radius. Football and Tennis balls are its examples.




ElliPse:
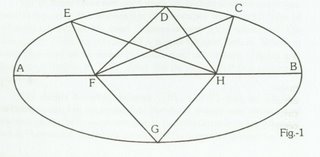
FIGURE 1

Ellipse is a figure of two dimensions and is like an egg. Fix two pins I
I
c


on the board, loop the string tightly stretched. The figure drawn is an
giijpge. In figure-I the points F and H where the pins arc fixed arc the
foci of the ellipse. Let the few points on the ellipse be AGBCD and E,
HC + CF = HD + OF = HE + EF = HA + AF = HG + GF =
HB+BF
It is commonly used in Astronomy.




Great Circle:
A great circle on the surface of the sphere is that circle whose
plane passes through the centre of the sphere. This circle divides the
sphere into two equal parts and its diameter passes through the centre
of the sphere. The circle made on the suface of an apple or an orange,
when they are cut from the middle, is a great circle.
In figure -2, C is the centre of the sphere and circle ABD is a great
circle, whose diameter ACB is passing through the centre C.
It is evident that great circles on a sphere are equal in magnitude.

Small circle:

A plane which docs not pass through the centre of the sphere
makes a small circle at the surface of the sphere. As such these circles
2Ve maller than a great circle and are called small circles. In fig - 2,
re e EHF ‘s a small circle as its diameter does not pass through the
cen~ee C.

Pole
:
A straight line drawn perpendicular to the plane of a great circle
and passing through the centre of the circle/sphere meets the sphere at
two points. These points are the poles of the great circle, one is above
the great circle and the other below it. In figure - 2, N and S are the
poles ofgreat circle ADB.

Properties of a great circle and poles:
(a) Great circles passing through the poles of a great circle are
called secondaries to the later circle. These secondaries cut the great
circle at right angles. In figure - 2, ADB is a great circle and its poles are
N and S. The great circles NLS and NMS arc secondaries and the angle
between them at L and M are right angles.

(b) Every great circle has two poles on the sphere in opposite
directions.

(c) A pole has only one great circle.

Axis:

Axis is a sfaaight line passing through the poles of a great circle
and its centre. In figure - 2, NCS is the axis of great circle ADB. in
Astronomy axis is a line around which a planet rotates.

Shape of the earth:
Earth is a spheroid like the orange. It is a bit flat on the poles.

Terrestrial equator:

Torroctrial oniiator is an imaoinarv oreat circle on the surface of
the earth which divides the earth in two equal hemi-spheres and it»
poles are in the centre of both the flat portions. It the sphere in iiguw -
2 is taken as earth, the circle ADB is the terrestrial equator N and S aw
· L. -i. TU„ rv\~innn ;Ihn„o thic oniiator is called the Northern heiTli-


sphere and the lower one as Southern hemi-sphere. NCS is the axis of ‘
the earth and C is its centre. ‘”

Terrestrial latitude:

lo find the position of a place on the earth, we require a set erf W
coordinates which are called latitude and longitude. I
Imagine small circles on the surface of the earth parallel to tbi~j
equator. The centre of all these circles will lie on the axis of the earth. 11*1
figure - 3, ACB is the diameter of the equator and NCS is the axis of ~»l
earth. M is a place on the earth. EMF is a small circle parallel to tf~i
equator. NMRS is a great circle which intersects the small circle at Mwell as the equator at R at right angles. Angle MCR (which is in a pl~l
perpendicular to the equator) is the terrestrial latitude of the place M. It
is the latitude of all places lying on the small circle EMF. The places
north of the equator have their latitudes between 0° to 90° (N) and
those in the southern hemisphere have their latitudes between 0° to 90°
(s).

Tropic of Cancer:

Tropic of Cancer is an imaginery line round the surface of the
earth parallel to the equator at an angular distance (latitude) of 23° 27’
(N). The sun shines at the head at mid-noon on about 21st June every
year. The sun enters sayana Cancer sign at this time. It is the reason of
naming this line as Tropic of Cancer.

Tropic of Capricorn :
When the sun goes maximum towards south on its orbit, it shines
above the head at places whose latitudes are 23° 27’ (S) and the
oedination of the sun becomes 23° 27’ (S). This small circle at 23° 27’
~ * “ tailed Tropic of Capricorn as the sun enters the sayan Capricorn
“rgn. This happens about 23rd of December every year.
4.11 Terrestrial Meridian:
‘ ~ Ihe Sreat circles on the surface of the earth and passing through
and other semi-the poles N and S are called terrestrial meridians of the
~iD”eS from which they are passing.

Prime Meridian:
To divided the earth between northern and southern hemispheres,
equator is there whose latitude is zero but for the starting point of
longitudes a problem arises as to which meridian be taken as the starting
point. In olden times the meridian passing through Ujjain used to be
taken as the Prime-meridian (zero degree meridian). Now a days the
meridian passing through the Greenwich observatory near London, is
considered as the Prime-meridian and the counting of longitudes starts
from this meridian.

Terrestrial longitude:
Angle of the arc intercepted at equator between the Prime-meridian
and the meridian of a place is known as terrestrial longitude of that
place. If the place is in the east of the Prime-meridian the longitudes arc
Eastward ‘E’ is suffixed after the degrees of longitudes and in case of
West W- is suffixed. Longitudes can be 0° to 180° (E) or 0° to 180° (W).
In figure -3, G is Greenwich and NGQS is the Prime meridian.
The longitudes of M is the angle QCR (E) as it is in the east of Greenwich.

Standard Meridian:
Every country/zone is not only spread from North to South but
from East to West also. The earth rotates around its axis from West to
East and completes one rotation in one day i.e. 24 hours. Therefore,
the sun appears moving from East to West daily. The places which are
in the east will have their mid-noon earlier than the places in the West,
resulting in the difference of local time. Local time of the places in the
cast will be ahead of the local time of the places in the West.
A problem arises that there will be difference of time at the places
east and west of a country/zone and the time schedule of trains,
Aeroplanes, TV. etc. cannot be framed. A person travelling from cast
to west or vice-versa will have to adjust his watch frequently due to
difference of local time. It was solved by finding out a way that in a
country/zone a meridian is chosen whose local time will be followed
throughout the country/zone and the wordly affairs are regulated
according to it This meridian is called the standard or central meridian
of the country/zone and its local time is said to be standard time of
that country/zone.
m

Celestial sphere:
If air is pumped into a balloon, its size increases gradually. Similariy
the earth may be projected into the space, the surface of this projected
sphere is known as celestial sphere. The centre of the earth is the centre
of the celestial sphere.
It is also defined as an imaginary sphere in the sky, centered on
the earth.

Celestial poles:
The place where the North and the South poles of the earth
meet the projected sphere (Celestial sphere) are the celestial North
and South poles. North pole is near Polaris and is directly above North
pole of the earth.

Celestial Equator:
Celestial equator is defined as the intersection of the earth’s
equatorial plane with the celestial sphere.
Celestial equator is a great circle on the celestial sphere, midway
between the poles. The place where the projected earth’s equator meets
the celestial sphere is the celestial equator.

Ecliptic:
The apparent annual path of the sun among the stars is known as
its orbit. When this orbit is expanded and the great circle formed by its
intersection with the celestial sphere is known as ecliptic.
Acually earth is revolving around the sun. The place where the
plane of earth’s orbit meets the celestial sphere is the ecliptic. The plane
of earth’s orbit is the plane of ecliptic. Mean angle between the planes
of ecliptic and celestial equator is 23° 27’. The angle between these two
plains varies from time to time and is called the obliquity of ecliptic.
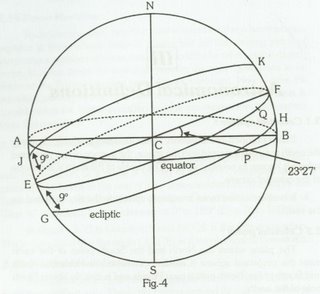
In figure - 4, APB is the celestial equator and EQF is the ecliptic.
C is the centre. The angle between their planes or say diameters i.e.
23°27’

Zodiac:
Our ancestors observed that the Moon and Planets were never at
a great angular distance from the ecliptic. They, therefore, conceived
an imaginary belt in the heavens extending about 9° on either side of
the ecliptic. This belt is known as zodiac. Moon and other planets are
found in this belt. Pluto sometimes goes out of this belt. The earth and
the sun are naturally in the middle of this belt which is ecliptic.
In figure - 4 of the celestial sphere, EQF is the ecliptic; JK and GH
are circles parallel to the ecliptic at a distance of 9° above and below it.
This belt JKHG is called as zodiac. In other words the space covered by
JEG moving round the celestial sphere is zodiac.
The definitions AS GIVEN ABOVE have been explained by a
diagram .

Celestial longitude:
The celestial longitude of a heavenly body at any time is the angle
of the arc measured along the ecliptic, from the first point of Aries to
the foot of the perpendicular drawn on the ecliptic from the heavenly body.